Source: Water Resources Research
To a casual onlooker, a winding river may elicit visions of canoeing, fishing, or swimming, but, for some scientists, flowing bodies of water bring to mind mathematics. Rivers can be treated as experiments in fluid dynamics, and researchers have long been interested in modeling how flowing water sculpts the land around it and vice versa.
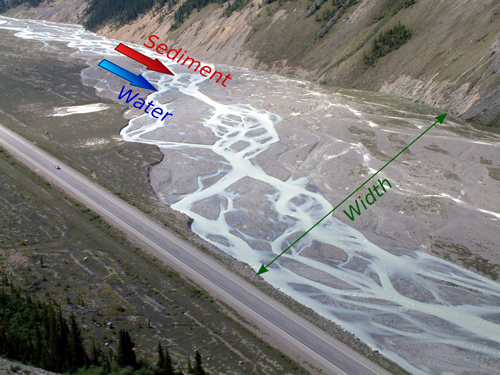
Braided rivers are composed of multiple channels separated by short slices of land between the river’s true edges; such rivers are especially difficult to model due to their variable boundaries and rapidly changing local topography. Here Redolfi et al. calculate a new, dimensionless indicator that reflects the shape of the riverbed and allows the team to derive other mathematical relationships important to modeling the flow of braided rivers.
The new indicator, called α, describes the shape of the riverbed when represented as a statistically averaged cross section. Some rivers’ cross sections are U-shaped—flaring out gradually from the base before shooting almost straight up near the banks; others are Y-shaped—wide at the top, but quickly narrowing to a very thin trough at depth; still others are somewhere in the middle—V-shaped with linearly slanted walls. Larger α values correspond to Y-shaped riverbeds, while lower ones produce U-shaped profiles. It’s important to note that α symbolizes an average representation of the river’s form over a period of uninterrupted distance known as a reach. Accordingly, all of the mathematical relationships revealed by the α indicator are also reach-scale in nature.
Generally speaking, a host of laboratory and field experiments suggest that braided rivers tend to have Y-shaped profiles, while single-thread rivers have more U-shaped profiles. The mathematics indicates that different profiles result from different hydrological regimes, bed material, and valley characteristics. When rivers have a high degree of confinement, meaning they occupy all the possible width that their surroundings permit—a river flowing through a thin, high-walled canyon, for instance—the profile moves toward a U shape.
When a value D, representing the depth of a river, is raised to the αth power, it produces a close approximation of the total width of the river covered by water. In a nonbraided river, this number would just be the bank-to-bank “wetted width,” but in braided rivers, the measurement becomes complicated because ridges of land also contribute to the total width.
The α indicator offers advantages over previous techniques because it does not depend on how much water is flowing through the river at any given time. Scientists can use the new metric to calculate other properties of braided rivers, such as how shear stress from the flowing water is distributed along a reach and the total volume of sediment particles moving through the river.
The new mathematics show that physical properties of braided rivers can be explained by a relatively simple power law—an insight that should allow scientists to more accurately model these bodies of water with less need for intensive field work to nail down specific river parameters. The authors note that the time and resources saved will be a definite boon to the field as water management becomes increasingly important in the face of climate change and growing world population. (Water Resources Research, doi:10.1002/2015WR017918, 2016)
—David Shultz, Freelance Writer
Citation:
Shultz, D. (2016), The mathematics of braided rivers, Eos, 97, https://doi.org/10.1029/2016EO053681. Published on 10 June 2016.
Text © 2016. The authors. CC BY-NC-ND 3.0
Except where otherwise noted, images are subject to copyright. Any reuse without express permission from the copyright owner is prohibited.

